[最も欲しかった] parabola congruent to y=x^2 842316

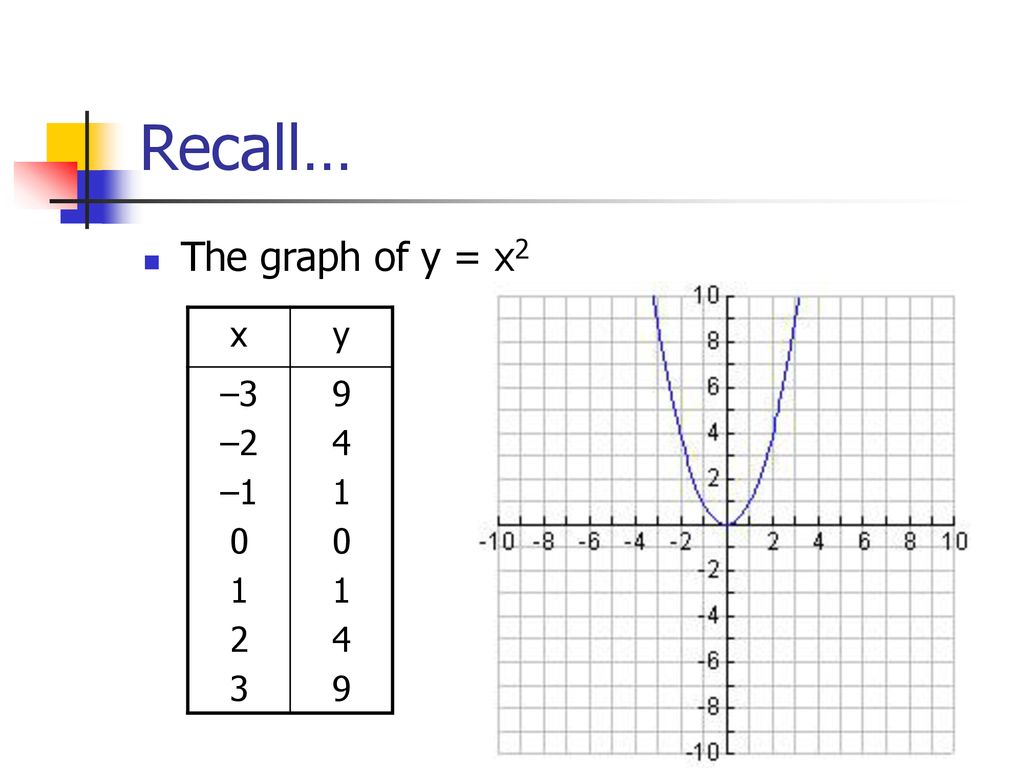
 Parabola described by y=2x^2 is narrower than the parabola described by y=x^2 Parabola described by y=2x^2 is narrower than the parabola described by y=x^2 Smaller the coefficient of x^2 wider the curveAnswer (1 of 4) The parabola has y axis as the axis Vertex is at V(0,0) The directrix y=2 is at distance 2 from the vertex V The Focus is at F=(0,2) Any point P(x,y) is at equidistance from y=2 and F PF^2=x^2(y2)^2 Distance from y=2 is y2 (y2)^2=x^2(y2)^2 y^22y4=x^2y^22y Consider a parabola P that is congruent to y=x^2, opens upward,and has its vertex at (2,4) Now find the equation of a new parabola that results if

Chapter 4 Quadratics 4 3 Using Technology To Investigate Transformations Ppt Download

Parabola congruent to y=x^2

Parabola congruent to y=x^2-Thanks so much in advance) Found 2 solutions by DrBeeee, josmiceliWrite the equation for each parabola with the given information mark each a) Congruent to y = 2x opens up, with a vertex of (51) > Congruent to y =(x 2), maximum vukue of 4, equation of asis of symmetry * = 2 10 Write the equation of the parabola with given vertex, if it passes through the given pointcz a) vertex (0,1), passing through (29) b)

Axis Of Symmetry Of A Parabola

Get an answer for 'Write the equation of a parabola with a vertex of (2,3) that opens downward and is congruent to y=1/3x^2 ' and find homework help for other Math questions at eNotesAbout Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsAdvanced Math questions and answers;

1 point 17) Which equation describes a parabola that opens downward, is congruent to y = x*2, and has its vertex at (0, 3)?Answer Correct option is B y = 0 The axis of symmetry of a parabola is a vertical line that divides the parabola into two congruent halves y2 2x = 0 y2 = −2x is the equation of a parabola It is in the form of y2 = 4ax So axis of parabola will be xaxis ( y = 0) as shown in the given figure Answer verified by TopprSubstitute the values of a a, d d, and e e into the vertex form a ( x d) 2 e a ( x d) 2 e 4 x 2 4 x 2 4 x 2 4 x 2 Set y y equal to the new right side y = 4 x 2 y = 4 x 2 y = 4 x 2 y = 4 x 2 Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k k

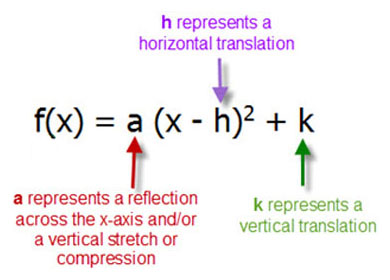
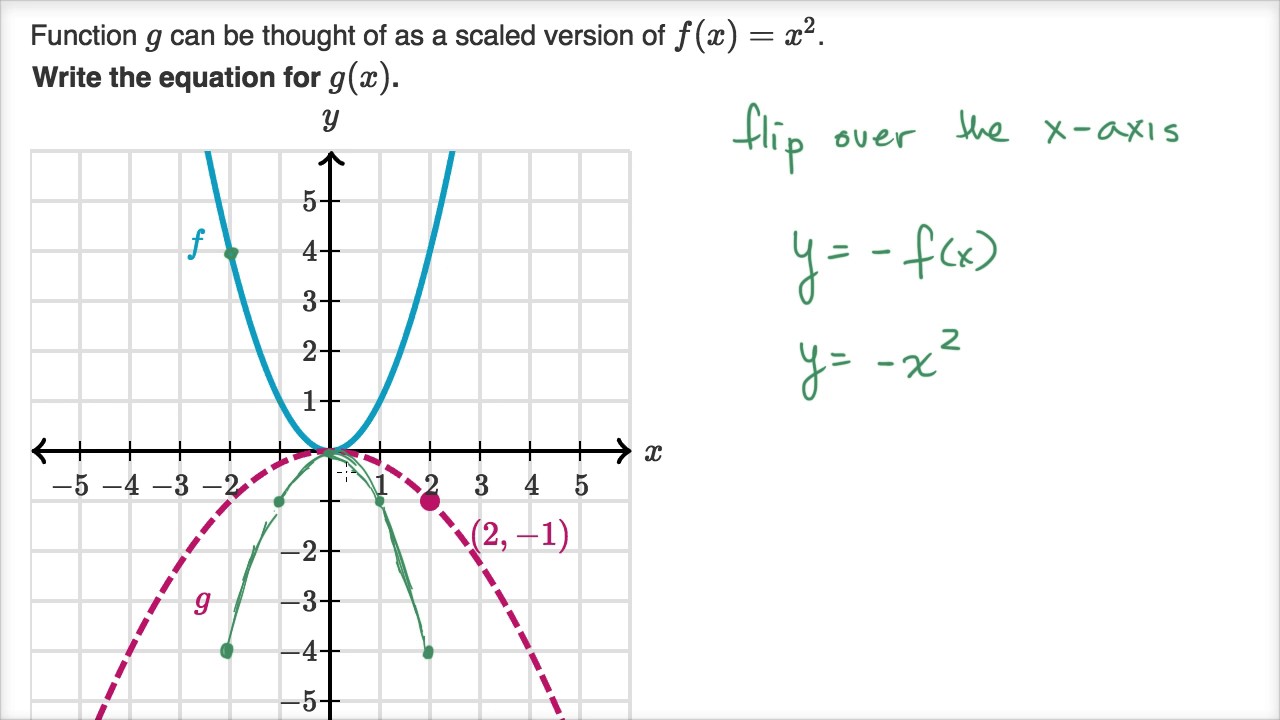
The equation of a parabola can be converted into the vertex form y = a(xh) 2 k, where h = xcoordinate of vertex, and k = ycoordinate of vertex Also, if a is positive, the parabola opens upward Also, if a is positive, the parabola opens upward Correct answer to the question Find the vertex of the parabola by completing the square x^26x8=y hmwhelpercom For example, when point P with coordinates (5,4) is reflecting across the Y axis and mapped onto point P', the coordinates of P' are (5,4)Notice that the ycoordinate for both points did not change, but the value of the xcoordinate changed from 5 to 5 You can think of reflections as a flip over a designated line of reflection

How To Graph A Parabola Y 4x 2 Socratic

Blessedmotherteresa Typepad Com

Write the equation with y 0 on one side y 0 = x 0 2 4 − x 0 5 This equation in ( x 0, y 0) is true for all other values on the parabola and hence we can rewrite with ( x, y) So, the equation of the parabola with focus ( 2, 5) and directrix is y = 3 is y = x 2 4 − x 5Arrow_forward Question Write an equation for a parabola that is congruent to the graph of y = x 2, opens downward, and has its vertex at (3,1) check_circle Expert AnswerY=x2–3 and compare it to † y=(x3)2 The analysis of responses attended to (1) common trends in explanations, and (2) attitudes towards perceived inconsistency RESULTS The fact that the shape of † y=(x3)2is a parabola that is congruent to the canonical parabola y=x2 was taken for granted by teachers and students alike The fact that the

Quadratic Functions Academic Support Center

Worksheet Key 6421 2 32 Am 9 2

Use traces to sketch the surface z = 4x2 y2 Solution If we put x = 0, we get z = y2, so the yzplane intersects the surface in a parabola If we put x = k (a constant), we get z = y2 4k2 This means that if we slice the graph with any plane parallel to the yzplane, we obtain a parabola that opens upward Similarly, if y = k, 2the trace is z = 4x k2, which is again a parabola that opensIf your parabola only crosses the xaxis at a single point, a, then that point will be the vertex of the parabola The equation of such a parabola will be mathy=(xa)^2/mathThe set of points (x, y) whose distance from the line y = 2 x 2 is the same as the distance from (2, 0) is a parabola This parabola is congruent to the parabola in standard form y = K x 2 for some K which is equal to

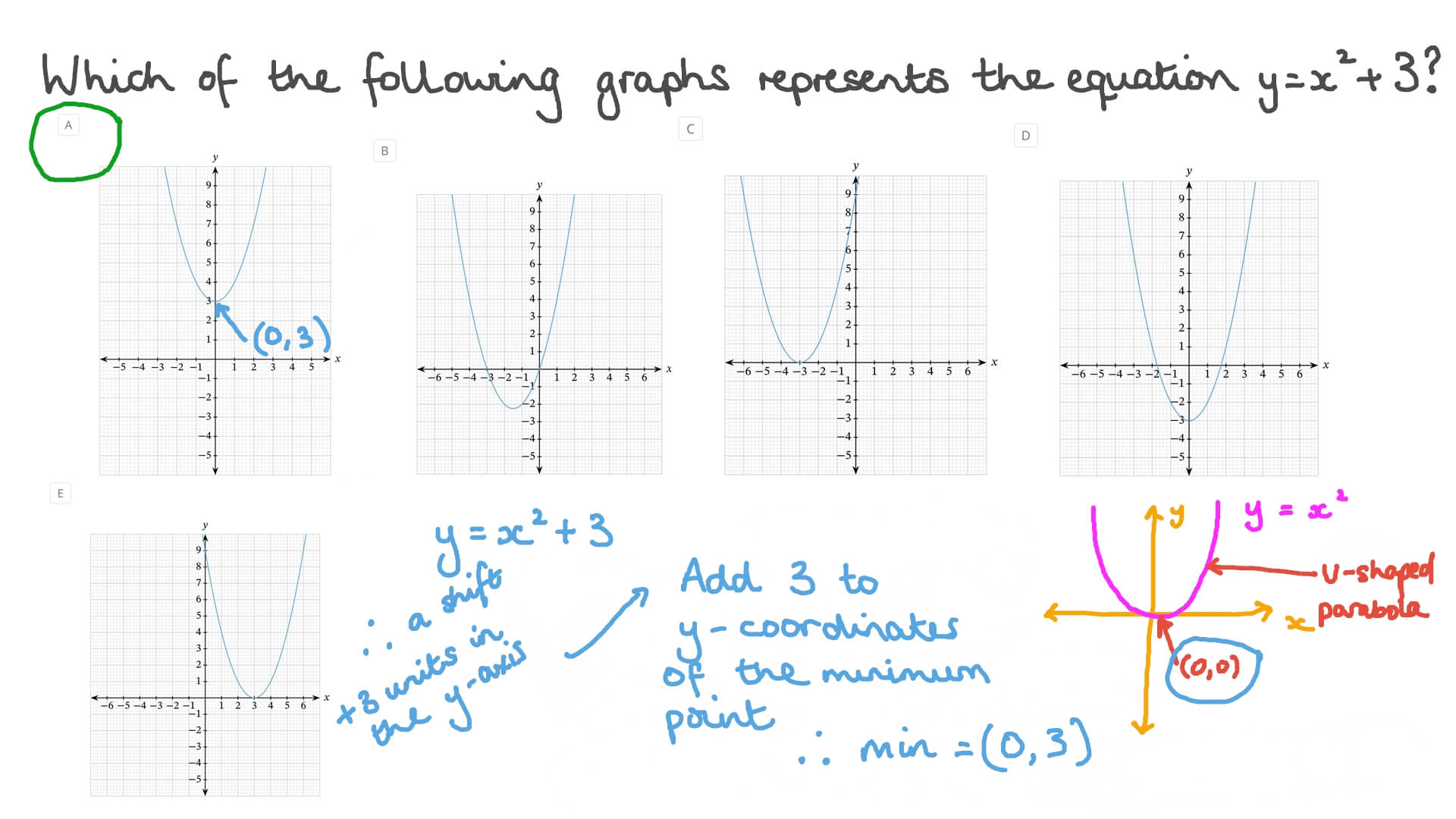
Question Video Identifying Graphs Of Quadratic Equations In Vertex Form Nagwa

Content Transformations Of The Parabola

Press J to jump to the feed Press question mark to learn the rest of the keyboard shortcuts You can't get any congruent triangles (which require three equal parts of a triangle to be equal), all you have are right angles as So p = 2 , q = 3 Given that congruent to y= 2x² and opens down congruent means has the same a value (same shape) of y= 2x² So a = 2, But it opens downSo a = 2 Substitute a = 2 , p = 2 , q = 3 in vortex form y = (2)(x (2))² 3 y = 2(x 2)² 3 The solution is y = 2(x 2)² 3Y = (x 3)2 1 y = – x2 3 B) y = (x – 3)2 y = x2 3

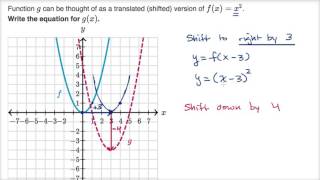
Shifting Parabolas Video Khan Academy

Engageny Org

What is the maximum vertical distance between the line y = x 6 and the parabola y = x2 for −2 ≤ x ≤ 3?Vertex\3x^22x5y6=0 vertex\x=y^2 vertex\ (y3)^2=8 (x5) vertex\ (x3)^2= (y1) parabolafunctionvertexcalculator enWrite an equation for a parabola that is congruent to the graph of y = x2, opens downward, and has its vertex at (3,1) close Start your trial now!

Content Transformations Of The Parabola

L2d2ii Html

Each parabola is affine congruent to P 0 y 2 = x Each ellipse or circle is affine congruent to E 0 x 2 y 2 = 0 Each hyperbola is affine congruent to H 0 xy = 1 proof of theorem AC2 Since two curves which are affine congruent to the same curve are affine congruent, we see that theParabola congruent to y = ax2 xintercepts at x = m and x = n (aka the "zeros" of the quadratic function) Regardless of the form, a parabola opens up when a > 0 and opens down when a < 0, with the axis of symmetry passing through the vertex of the parabolaWe can find the parabola's equation in vertex form following two steps Step 1 use the (known) coordinates of the vertex, ( h, k), to write the parabola 's equation in the form y = a ( x − h) 2 k the problem now only consists of having to find the value of the coefficient a Step 2 find the value of the coefficient a by substituting

Axis Of Symmetry Of A Parabola

What Are The X Intercepts And The Coordinates Of The Vertex For The Parabola Y X 2 4x 12 Quora

To find the endpoints, substitute x = 6 x = 6 into the original equation ( 6, ± 12) ( 6, ± 12) Next we plot the focus, directrix, and latus rectum, and draw a smooth curve to form the parabola Figure 7 Try It Graph y2 =−16x y 2 = − 16 x Identify and label Consider a parabola P that is congruent (has the same shape) to y=x^2 , opens upward, and has vertex (2,3) Now find the equation of a new parabola that results if P is Compressed to a factor of 1/2 Translated 2 units to the left Translated 3 units up Reflected in the xaxis and translated 2 units to the right and 4 units downQuestion Find the equation of the parabola with vertex (4,4), the parabola opens upwards and is congruent to y=1/2x^2 Can you please help me?

1

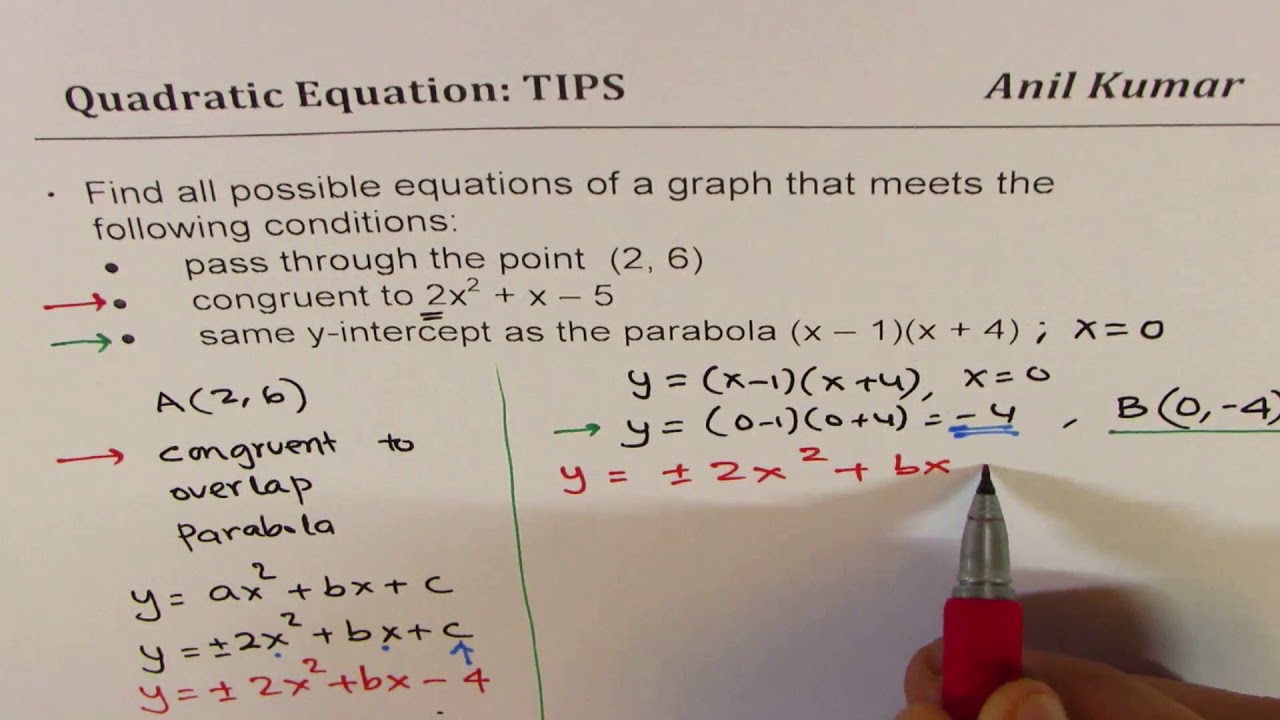
Quadratic Equation Congruent To Parabola Pass Through Point With Y Intercept As Another Curve Youtube

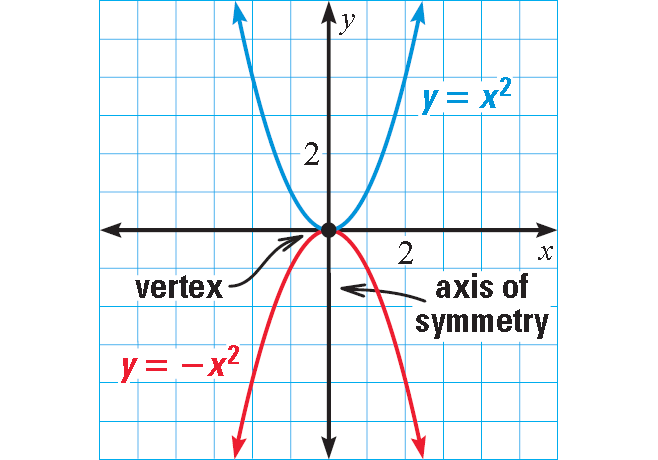
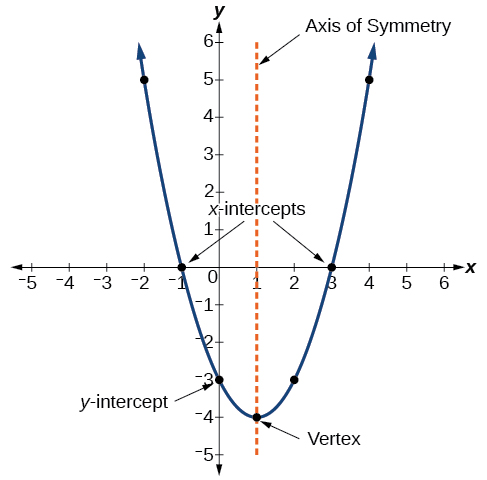
Axis\(y3)^2=8(x5) directrix\(x3)^2=(y1) parabolaequationcalculator y=3x^{2} en Related Symbolab blog posts Practice Makes Perfect Learning math takes practice, lots of practice Just like running, it takes practice and dedication If you wantEvery parabola has an axis of symmetry which is the line that divides the graph into two perfect halves On this page, we will practice drawing the axis on a graph, learning the formula, stating the equation of the axis of symmetry when we know the parabola's equation y = a(x – h) 2 k, where (h, k) The axis of symmetry of a parabola is a vertical line that divides the parabola into two congruent halves The axis of symmetry always passes through the vertex of the parabola The x coordinate of the vertex is the equation of the axis of symmetry of the parabola

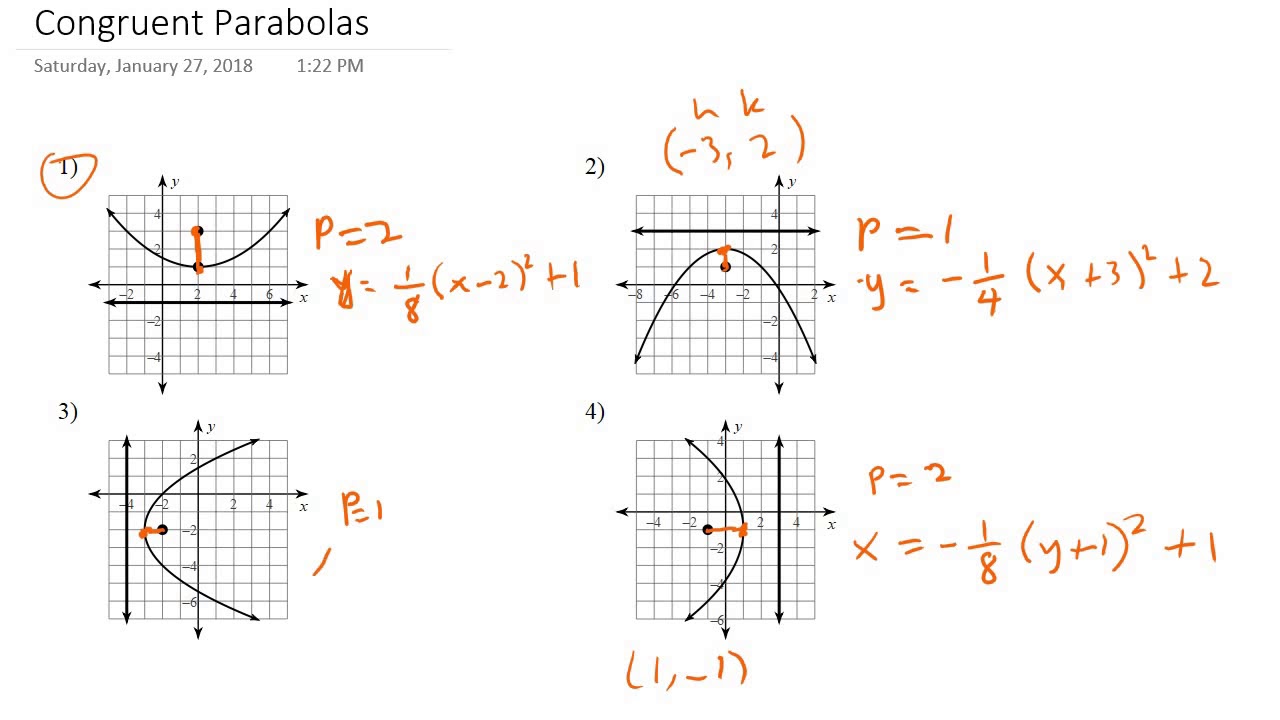
Congruent Parabolas Youtube

Solved Which Parabolas Shown Below Are Congruent To The Chegg Com

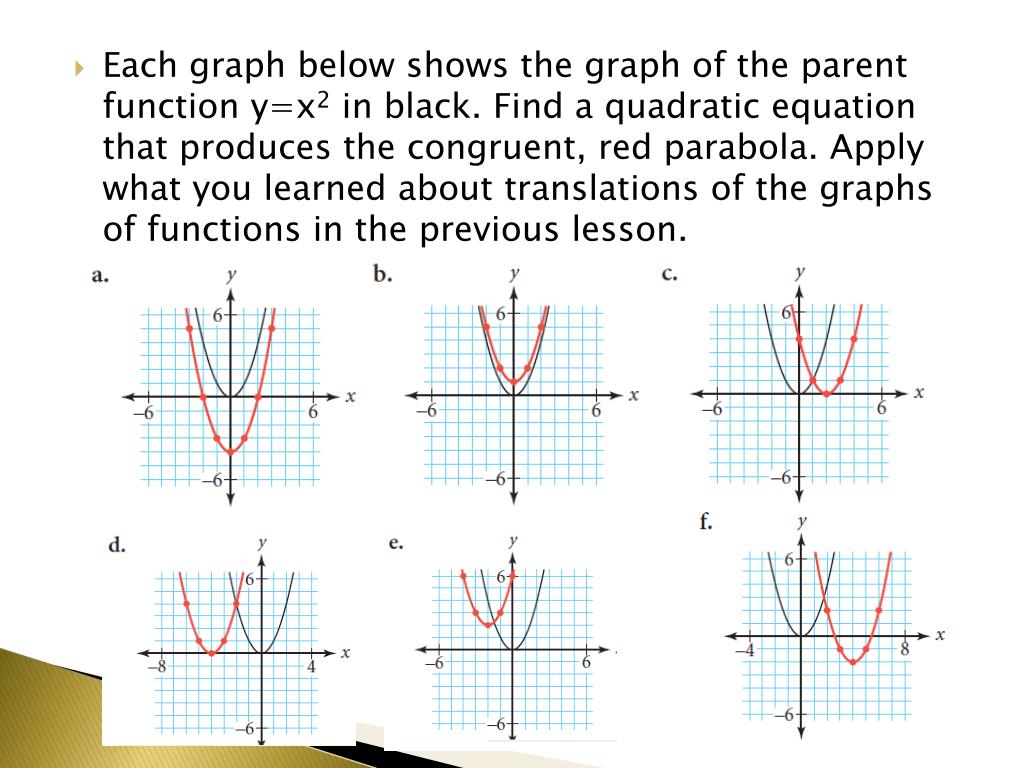
11 The graph below shows two congruent triangles, ABC and A'B'C' Which rigid motion would map ABC onto A'B'C'?1 start by plotting the vertex (if the parabola is congruent to y=x(squared) 2 plot the second point by moving left one unit and up one unit from the last point 3 plot the third point by moving left one unit and up three units from the last point 4 plot mirrored points to get the right half of the parabola•Consider a parabola that is congruent to y = 2 x, and with vertex (2, ‐4) Find the equation of a new parabola that results if it is (a) Translated 6 units down (b) Translated 5 units left (c) Translated 6 units up and 5 units right

The Axis Of A Parabola Is Along The Line Y X And The Distance Of Its Vertex And Focus From The Origin Are Sqrt 2 And 2sqrt 2 Respectively If Vertex And Focus Both

Quadratic Functions Academic Support Center

Y = − x 2 y = x 2 y = − x 2 y = x 2 Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k k a = − 1 a = 1 h = 0 h = 0 k = 0 k = 0 Since the value of a a is negative, the parabola opens down Opens Down Find the vertex ( h, k) ( h, k)Answer to 1 point 17) Which equation describes a parabola that Math;Y = 2 x2 4 x í 6 62/87,21 Graph the ordered pairs, and connect them to create a smooth curve The parabola extends to infinity The axis of symmetry is the line that goes through the vertex and divides the parabola into congruent halves It is located at x = ±2 Find the yintercept

Exploring Properties Of Parent Functions Part A Introduction

1

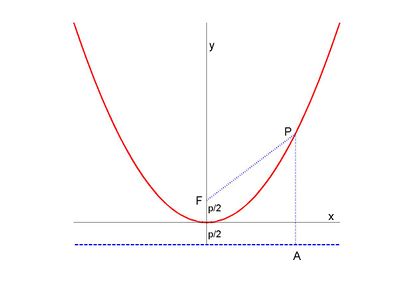
View HW Day 2 congruent parabolas (1)pdf from MATH 1101 at Syosset High School Name _ Day 2 – congruent parabolas 1) Write the equation of the parabola whose directrix is x = 2Parabolic mirrors concentrate incoming vertical light beams in their focus We show this Consider in figure 2 the arbitrary vertical light beam B (blue, parallel to the yaxis) that enters the parabola and hits it at point P = (x 1, y 1)The parabola (red) has focus in point FThe incoming beam is reflected at P obeying the wellknown law incidence angle is equal to angle of reflectionTherefore the "p" translates the parabola horizontally across the xaxis, and the "q" translates the parabola vertically across the yaxis The "p" value also represents our axis of symmetry, the line that divides the graph into two congruent parts, so that the graph on one side is the mirror image of the graph on the other side

What Is The Equation For A Parabola That Is Congruent To The Graph Of Y X Squared Opens Downward And Has Its Vertex At 3 1 Quora

Solving Quadratic Equations By Graphing

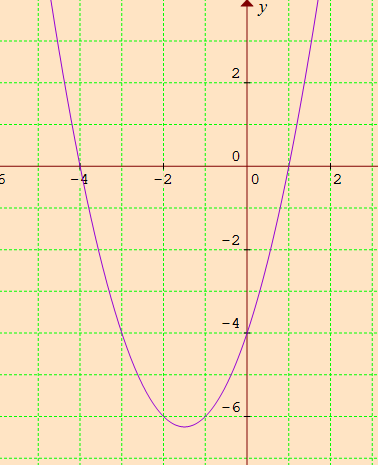
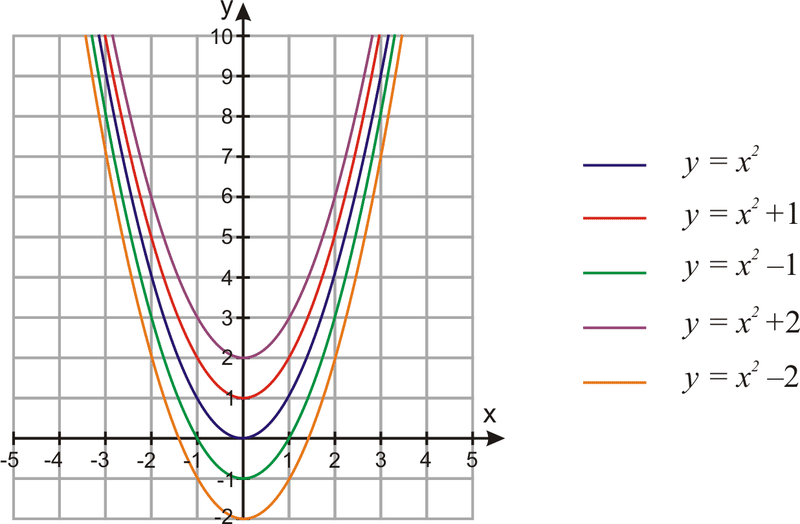
 Six congruent copies of the parabola $y = x^2$ are arranged in the plane so that each vertex is tangent to a circle, and each parabola isThe equation of this new parabola is thus y = x 2 9 The vertex of this parabola is now (0, 9), but it has the same axis of symmetry Similarly, the basic parabola becomes y = x 2 − 9 when translated down 9 units, with vertex (0, 9) We can confirm thisGenerally, only the higher level of high school maths contain locus questions (especially year 11) As discussed earlier, we have to deal with the locus form of quadratics, which is given as $$(xh)^2=±4a(yk)$$ We have already seen that (h,k) represent the vertex of the quadratic

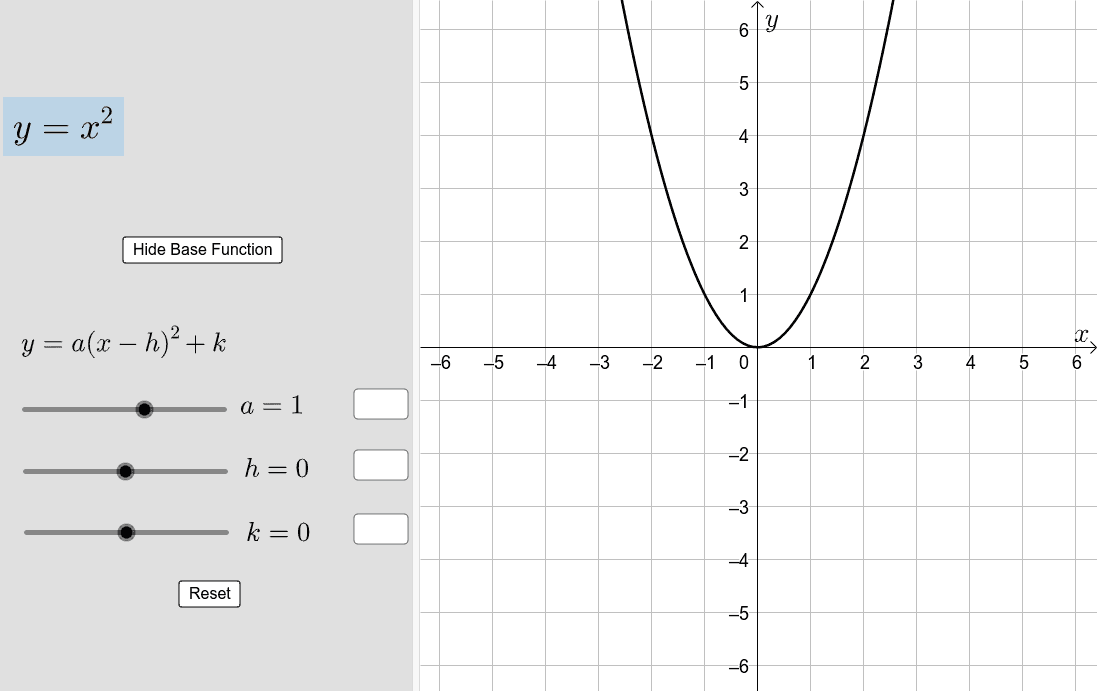
Transforming The Graph Of Y X Geogebra

Parabola Parent Function Mathbitsnotebook A1 Ccss Math

 In δabc shown below, ∠bac is congruent to ∠bca triangle abc, where angles a and c are congruent given base ∠bac and ∠acb are congruent prove δabc is an isosceles triangle when completed (fill in the blanks), the following paragraph proves that line segment ab is congruent to line segment bc making δabc an isosceles triangleConsider the parabola y = x 2 Since all parabolas are similar, this simple case represents all others Construction and definitions The point E is an arbitrary point on the parabola The focus is F, the vertex is A (the origin), and the line FA is the axis of symmetry The line EC is parallel to the axis of symmetry and intersects the x axisFirst week only $499!

The Graph Of Y X2 6x 9 Is Shown Below What Is The Solution Set For Brainly Com

Content Transformations Of The Parabola

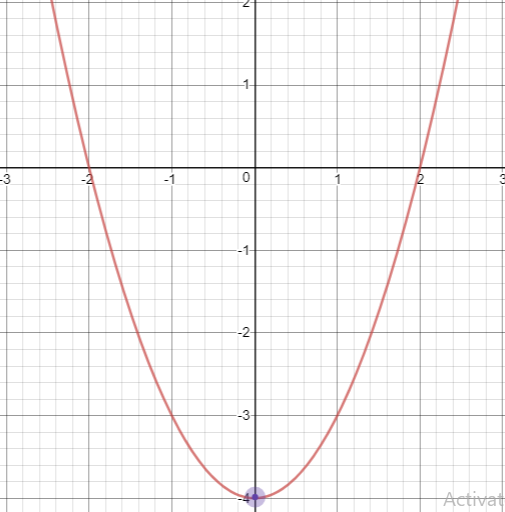
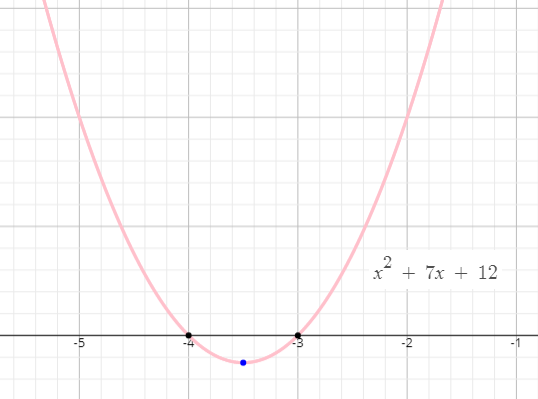
Since it is so simple to find the yintercept (and it will probably be a point in my Tchart anyway), they are only asking for the xintercepts this timeTo find the xintercept, I set y equal 0 and solveFind the xintercepts and vertex of y = –x 2 – 4x 2;A parabola is congruent to y=x^2 and has xintercepts 1 and 5 determine the coordinates of the vertex Answer by Fombitz() ( Show Source ) You can put this solution on YOUR website!

Transformations Of Functions Ck 12 Foundation

Find The Centroid Of The Region Bounded By The Parabola Y X 2 The Line X 2 And The X Axis Study Com

1) a rotation of 90 degrees counterclockwise about the origin 2) a translation of three units to the left and three units up 3) a rotation of 180 degrees about the origin 4) a reflection over the line y =xSuppose the parabola with equation y = 2x^2 undergoes the translation T2,3 Find an equation for its image Vertex form is y k = a(x h)^2 Also given is Th,k, with h = 2 and k = 3 Also given is that a = 2 in the expression 2x^2 Now fill in a, h and k with the given numbers y 3 = 2(x 2)^2 > and there's the answer

Consider A Parabola P That Is Congruent To Y X 2 Opens Upward And Has Vertex 0 0 Find The Brainly Com

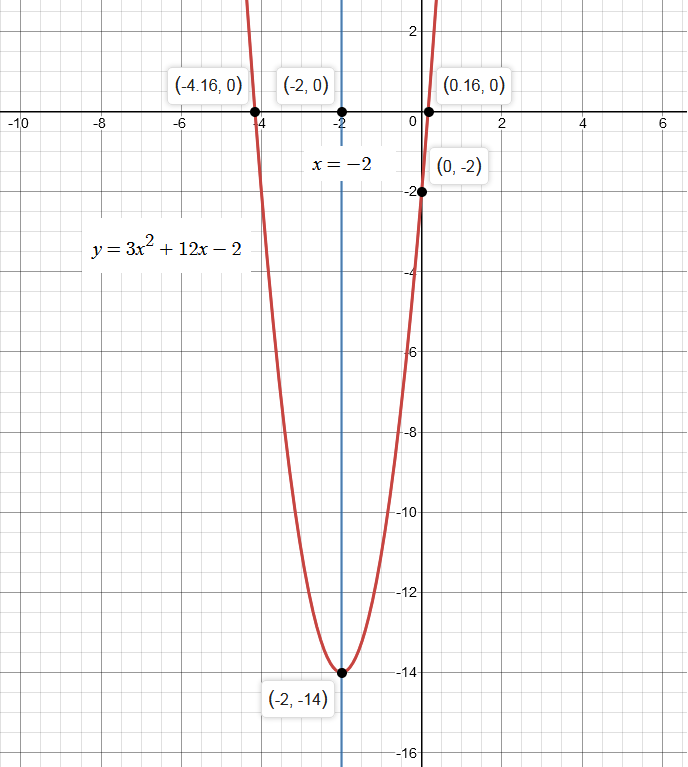
What Is The Axis Of Symmetry And Vertex For The Graph Y 3x 2 12x 2 Socratic

L2d2ii Html

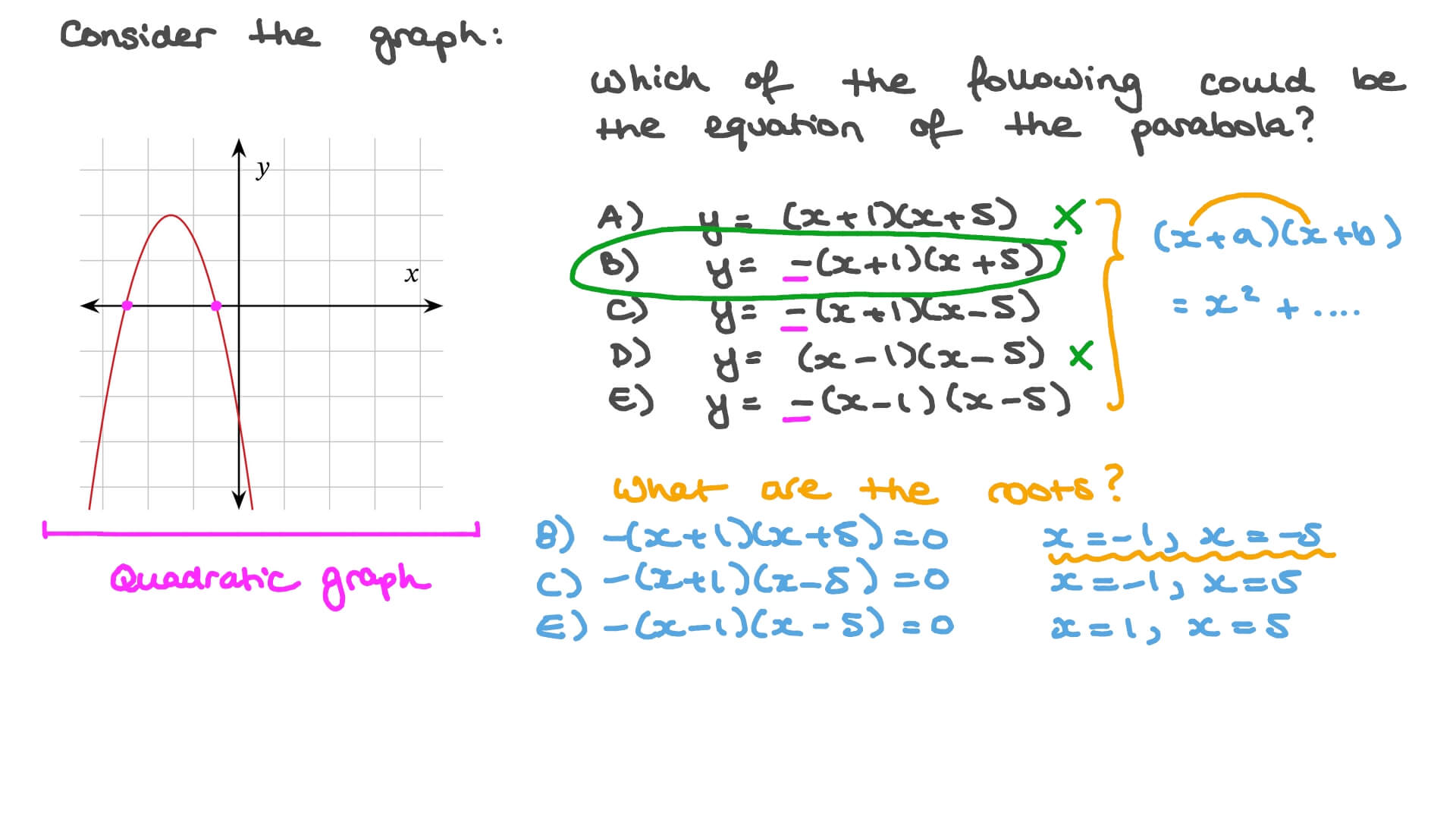
Question Video Equations Of Parabolic Curves Nagwa

Parabola Parent Function Mathbitsnotebook A1 Ccss Math

Algebra Ii Module 1 Topic C Lesson 35 Student Version

How To Find The Minimum Or Maximum Value Of A Function In Vertex Form

Algebra 2 Module 1 Lesson 35 Video Youtube

Graphing Quadratic Functions Parabola

Pubs Lib Umn Edu

Solving Quadratic Equations By Graphing Examples

Unique Quadratic Equation In The Form Y Ax 2 Bx C

Solved Write An Equation Of A Parabola That Has A Vertex 3 8 Opens Downward And I Congruent In Shape To The Graph Of Y 5x2 1 The X Interce Course Hero

Attributes Of Quadratic Flashcards Quizlet

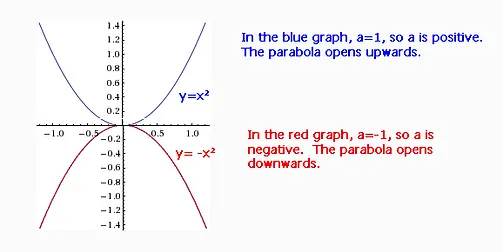
Vertex Form Chapter 4 Quad Relationships

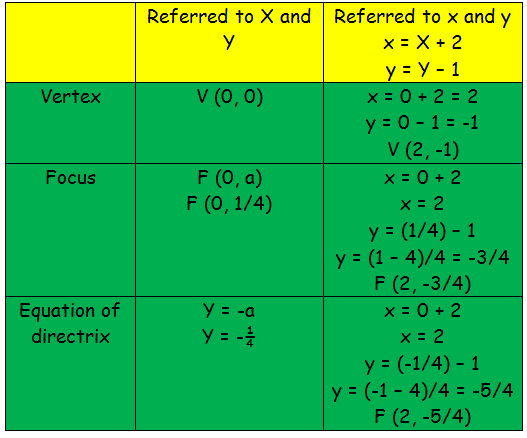
How To Find Focus Directrix And Vertex Of Parabola

Congruent Parabolas Youtube

Exploration Of Parabolas

1

Content Transformations Of The Parabola

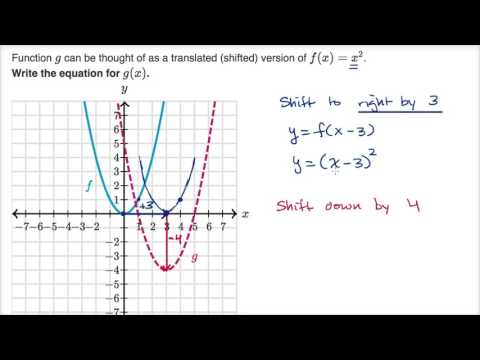
Shifting Parabolas Video Khan Academy

Parabola Wikipedia

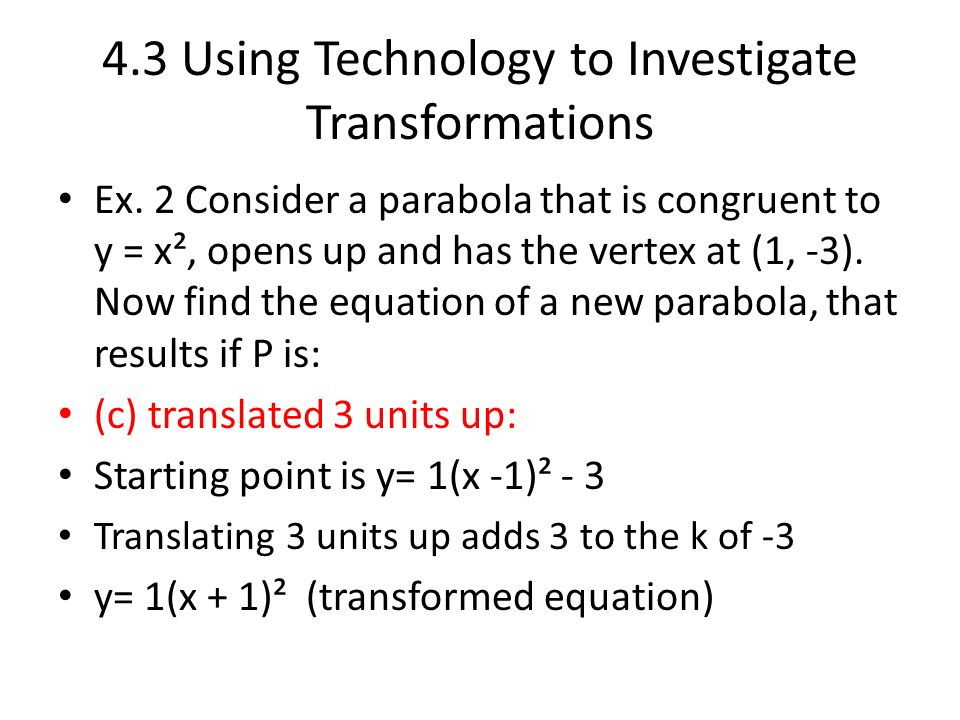
Chapter 4 Quadratics 4 3 Using Technology To Investigate Transformations Ppt Download

Engageny Org

Axis Of Symmetry Of A Parabola

Blessedmotherteresa Typepad Com

Algebra1 Graphing Y Ax 2 Youtube

5 2 Quadratic Functions Mathematics Libretexts

Vertical Shifts Of Quadratic Functions Ck 12 Foundation

Math Spoken Here Classes Quadratic Equations 3

Quadratic Function

What Is The Equation Of A Parabola That Opens Upward And Has X Intercepts At 2 0 And 6 0 Homeworklib

Plot A Graph For The Equation Y X 2 4x 1

Transformations Of Functions Ck 12 Foundation

Chap 7 The Parabola Pdf Pdf Quadratic Equation Geometry

Graphing Quadratic Functions

Ppt Analyzing Parabolas Powerpoint Presentation Free Download Id

Chapter 4 Quadratics 4 3 Using Technology To Investigate Transformations Ppt Download

Resourceaholic Tricks And Tips 3 Quadratics

Exploration Of Parabolas

Consider The Parabola Whose Equation Is Y X 2 4x And The Line Y 2x B Then Whichfollowing Is Are Correct

In The Figure Below The Equation Of The Solid Parabola Is Y X 2 21 And The Equation Of The Line Is Y x Determine The Area Of The Shaded Region Set Up The Integral That

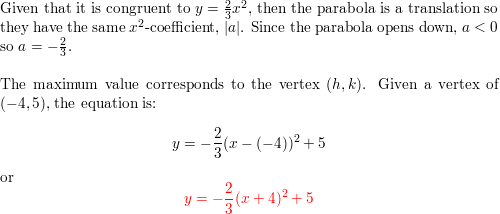
Write The Equation For Each Parabola In The Form Y A X H 2 K With The Given Information Congruent To Y 2 3x 2 Opens Down And Has A Maximum Value At 4 5 Homework Help And

Parabola Sketching Y 2x 2 4x 6 From The Parabola Translation Download Scientific Diagram

Axis Of Symmetry Of A Parabola

9 1 Identifying The Characteristics Of And Graphing

How Do You Graph Y X 2 2

Quadratic Function

The Parabola

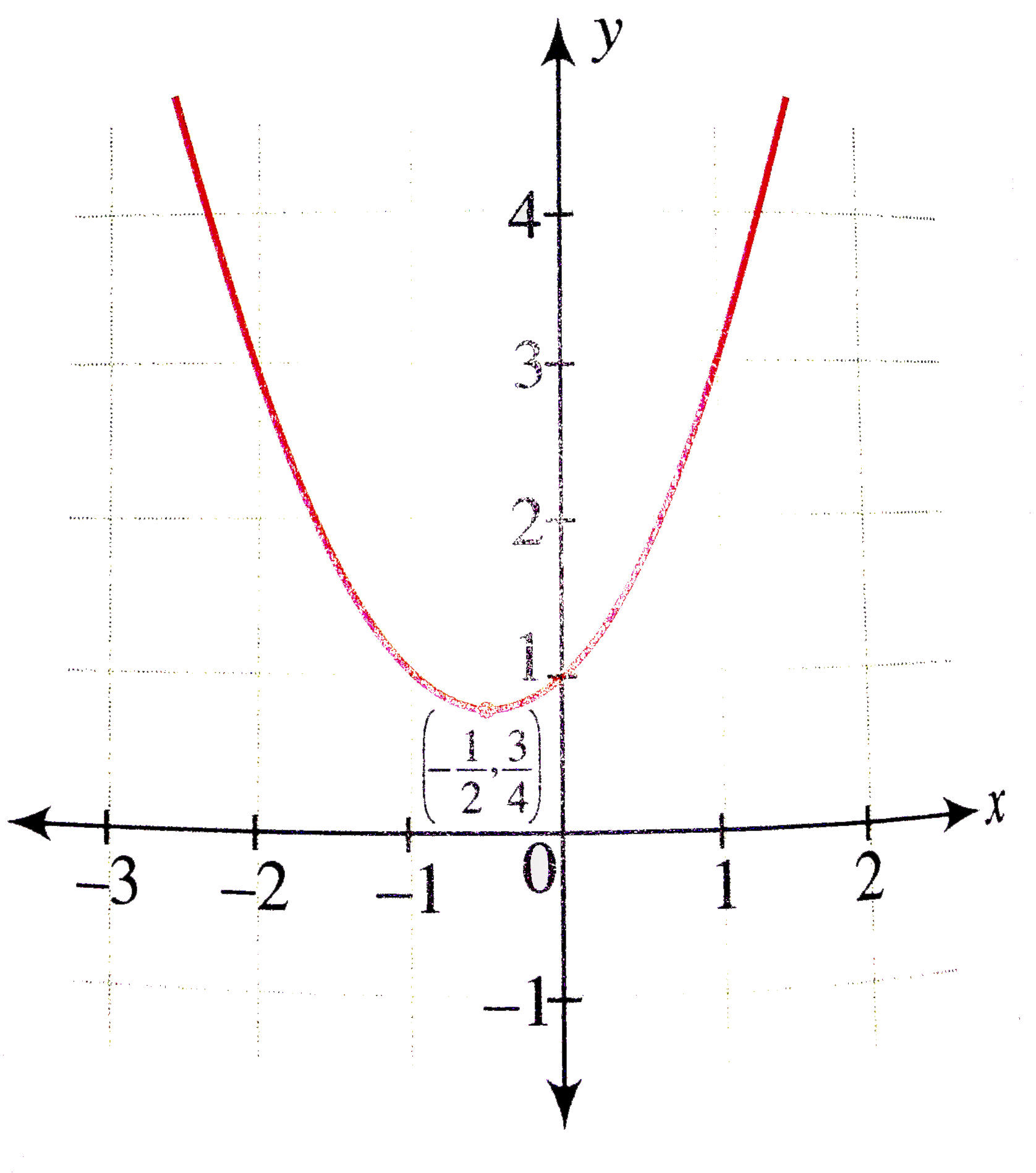
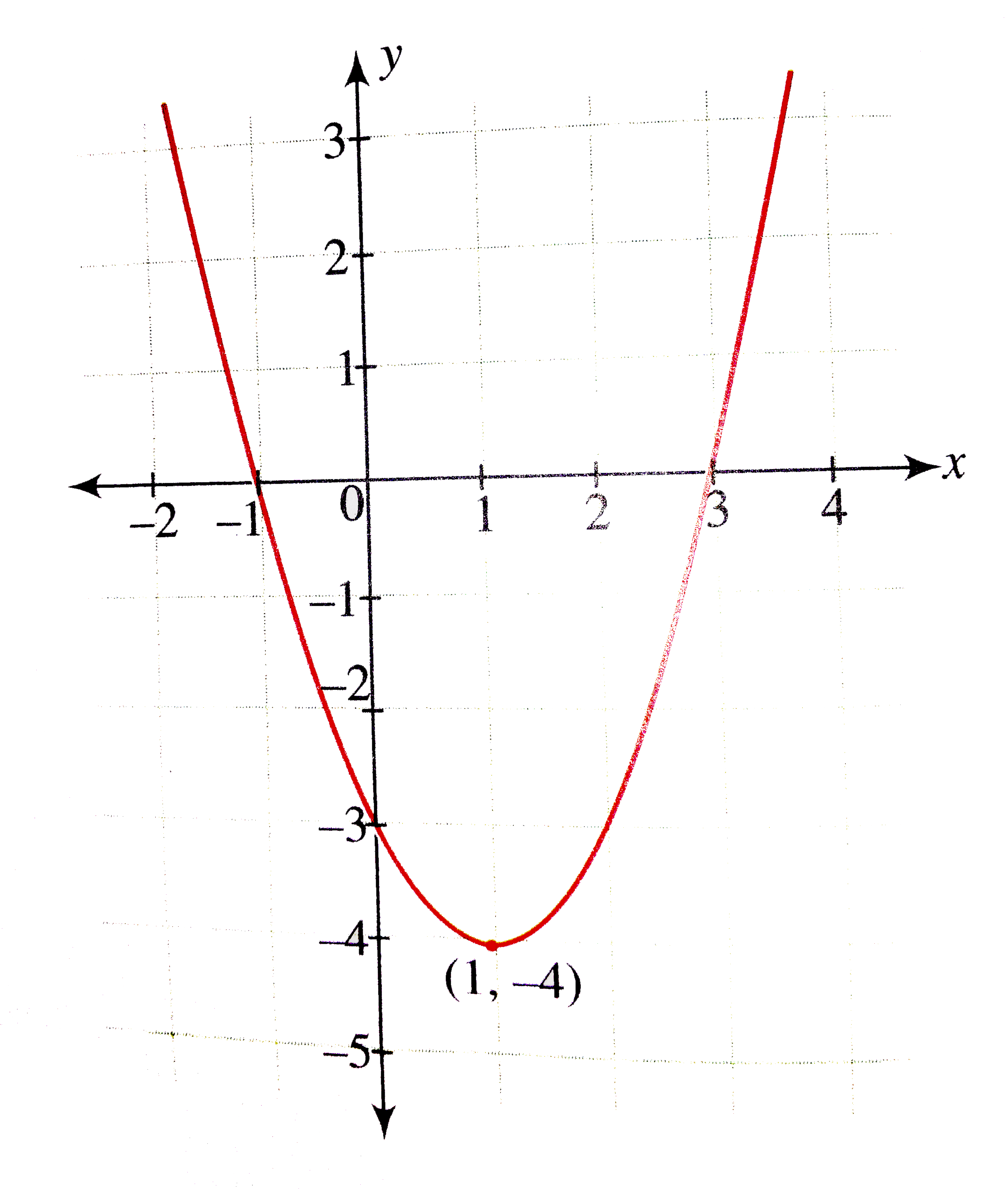
Draw The Graphs Of Following Quadratic Functions I Y X 2 X 1 Ii Y X 2 2x 3 Iii Y 2 X X 2 Iv Y X 1 X 2

Graphs Of Parabolas Vertex Form Pdf

Untitled Document

Ppt Translating And The Quadratic Family Powerpoint Presentation Free Download Id

1

Example 1 Write A Quadratic Function In Vertex Form Write A Quadratic Function For The Parabola Shown Solution Use Ver Quadratics Quadratic Functions Parabola

Consider A Parabola Math Y X 2 Math The Line That Goes Through The Point 0 3 2 And Is Orthogonal To A Tangent Line To The Part Of Parabola Math Y X 2 Math With Math X 0 Math Is Math Y Ax 3 2 Math What

Solved 2 Marks 4 If The Point 2 7 Is On The Parabola Y Chegg Com

Solved 1 Point 17 Which Equation Describes A Parabola That Chegg Com

Scaling Reflecting Parabolas Video Khan Academy

Graphing Quadratic Functions Lesson Article Khan Academy

Quadratic Function

Parabola Knowino

Content Transformations Of The Parabola

The Parabola Shown Is Congruent To Y X 2 A What Are The Zeroes Of The Function B Write The Equation In Factored Form Quora

Parabola Wikipedia

Solved Write The Equation Of The Parabola That Opens Down Has A Vertex V 1 5 And Is Congruent To Y I332 Answer In The Form 3 A C H 2 I Course Hero

What Is The Equation For A Parabola That Is Congruent To The Graph Of Y X Squared Opens Downward And Has Its Vertex At 3 1 Quora

Solution Can You Help Me With This Problem A Parabola Is Congruent To Y X 2 And Has X Intercepts 1 And 5 Determine The Coordinates Of The Vertex

Lesson 4 2 Graphing Parabolas Using Transformations Ppt Download

What Is The Equation For A Parabola That Is Congruent To The Graph Of Y X Squared Opens Downward And Has Its Vertex At 3 1 Quora

Whiteplainspublicschools Org

The Point 5 4 Lies On The Graph Of A Quadratic Function Whose Axis Of Symmetry Is X 2 What Is Another Point On The Graph Quora

Find The Centroid Of The Area Bounded By The Parabola Y 4 X 2 And The X Axis Study Com

Solution Find The Equation Of The Parabola With Vertex 4 4 The Parabola Opens Upwards And Is Congruent To Y 1 2x 2 Can You Please Help Me Thanks So Much In Advance

Draw The Graphs Of Following Quadratic Functions I Y X 2 X 1 Ii Y X 2 2x 3 Iii Y 2 X X 2 Iv Y X 1 X 2

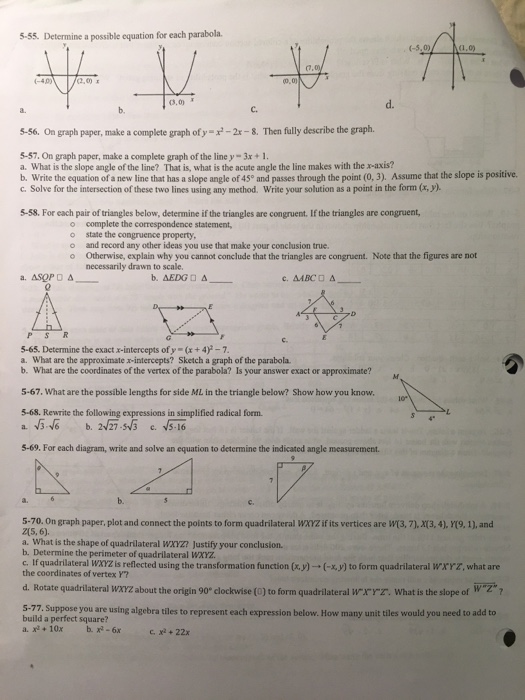
Solved Determine A Possible Equation For Each Parabola On Chegg Com